This web page is about teaching the basic concept of proportion, together with the related concept of probability. This is just a beginning. But I spent a long time thinking about how to teach this as a concept without any answers, so I am somewhat proud of this beginning.
The Two Faces of Fractions: Numbers and Proportions
I spent several months recently trying to understand fractions. To the best of my understanding, there are three distinct meanings. The first is as a process -- if we want to indicate that 13 should be divided by 7, we can write 13/7. The second is as a number. For example, 13/7 is the number produced by dividing 13 by 7.
It turns out that we usually indicate numbers by process, so these two meanings are closely related.
The third meaning is, in a way, completely different -- as a proportion. Suppose the proportion of girls in a classroom is 2/3. This does not say how many girls are in the classroom. To try to help a student understand the concept of 2/3 (or why 2/3 is the same oa 4/6), the student might be shown a circle divided into three parts, with two of the parts shaded in. This fits the concept of 2/3. It has little to no attachment to the process or the actual number 2/3. (To connect it to the number 2/3, you would have to say that the area of the circle is 1. But that won't be important for anything else you do with the circle, and it certainly is not a part of the pretty picture of a 2/3 shaded circle that is supposed to represent 2/3.)
Proportions are always numbers from 0 to 1. Essentially, you can attach any meaning to higher or lower numbers. This is unlike the other two meanings of fractions.
Percentages & Probabilities
A percentage is often explained as being a number. For example, 50% corresponds to the number .50; 374% corresponds to the number 3.74. Technically, this is true.
However, it misdirects the usually use of percentages. Percentages are usually used to express proportions. As such they are most meaningful in the range from 0% to 100%. I know they can be used outside this range, but it is not as common and (at least in the examples I can think of), not as easily understood. So we talk about 54% of the class being girls, or 2% of the products being defective, or 99% of the families owning a television.
A probability is just a proportion, and again it is a number between 0 and 1 and often represented with percentages. Suppose a bag contains 2 black marbles and 1 white marble. Then the proportion of black marbles is 2/3, and the probability of choosing a black marble is 2/3. I suspect that beginning students would rather represent a probability as a ratio, which is what happens in many betting games. So the odds of choosing a black marble from that bag are 2 to 1. However, these numbers are not easy to work with in calculating the probability of two events.
Parenthetically, the concept of average, from statistics, is very much related to proportion -- both talk about the overall tendency of a set independent of how many items are in the set. And of course a statistical test can be on a proportion as easily as on an average.
Meaningfulness
You have to realize that in many situations involving inferences, we care about proportions. Suppose you are buying a lottery ticket. Do you want to know how many winning tickets there are, or do you want to know what proportion of tickets are winning tickets. If you catch a disease, do you want to know how many people have died from the disease, or what proportion of people catching the disease have died from it?
A Diagnostic
Consider the drawing below. It is from my "Same Problems." The students have to discover how the things on the left are all the same, yet different from the things on the right. You can go that web page for examples of those problems, and you should use some of those problems to familiarize your students with the problem format before giving them this problem.
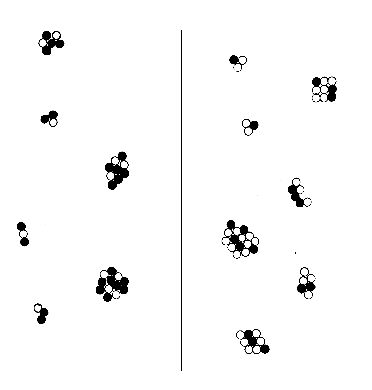
The answer, in rough terms, requires the notion of proportion. I had one third grader get the answer, though it was not easy; a third-grader and a kindergartner could not get the answer. So this apparently is not always an easy problem. If a student cannot get this problem, the student probably does not have the concept of proportion.
Teaching the Concept of Proportion
Honest, I thought weeks about this: What is the problem that proportion is the answer to? (Of course, it had to be a problem they could solve.) Finally I thought of this, taking a model from the field of probability. They are given a choice of two bags. One contains 3 black marbles and 1 white marble. The other contains 5 black marbles and 10 white marbles. If they want to select a black marble, which bag should they choose from? My five-year could solve this problem, so I guess at some young age they build enough of a mental model to solve this problem.
In terms of practical details, I used bowls instead of bags, and I used checkers instead of marbles. I offered my 5-year-old a dime for each time she selected a black marble, but she seemed more interested in getting the black marble than the dime and actually gave them back to me until I suggested she could put them in her piggy bank.
The question then is, why are they taking a black marble from the bag with the fewest black marbles? I just ask them to explain their choice.
This question has many different possible answers. Your problem is a student who cannot think of answers. My kindergartner could think of several.
First she said that many people would focus on the number of black marbles, but she choose the bag with the fewest white marbles. So I gave her a choice, one bag with 20 black marbles and 5 white ones, and another with 3 white ones and 1 black one.
Then she wanted the difference between black and white to be as large as possible. Another nice hypothesis. I gave her a choice between 20 black and 12 white versus 4 black and 1 white.
She chose correctly, and I think she noticed the contradiction between her choice and her rule. But she had no more hypotheses to offer.
Finally, her choice was a bag with 2 black marbles and 1 white marble and another bag with 4 black marbles and 2 white marbles. I was hoping she would see them as the same, but no luck. So that day did not finish with the answer. It's just my intuition that she is now more likely to solve the proportion problem.
Well, I did this with bright third-graders and bright sixth-graders. Two of the third-graders, who could not solve the "Same" problem involving proportions, were about the same as the kindergartner. However, they immediately said the problem involved "chance." The third kindergartner, who could do the "Same" problem involving proportions, did better in the short time she was tested, but did not spontaneously get it right quickly.
The story was different for sixth-graders, perhaps because they are more comfortable with division and fractions. One immediately saw the answer in terms of ratios; a second quickly saw the answer in terms of fractions (proportions). The third went the same route as the third-graders, only much more quickly, and immediately latched onto fractions when they were suggested to him. I explained that fractions and ratios were both used to represent
Inferential Statistics
Inferential Statistics is normally considered an advanced topic, taught in college. But in these exercises, the students are just inferring the proportion, which is taken for granted in a college statistics course. (I have not yet done this exercise.)
Anyway, the exercise is this. The teacher has a bag with marbles. The teacher reaches in, selects a random marble, shows it, records the color, and puts the marble back in. This is repeated. After a while, the question is this: What does the student think is in the bag?
Part of this assignment is guessing how many marbles are in the bag. If the teacher can avoid making noise, this is essentially impossible for the students to do -- the results of drawing, say 2/3 black, are consistent with any 2 to 1 ratio of black to white. (Of course, if an answer is 1/10, there must be at least 10 marbles in the bag. It would be great if the students could realize that they cannot know the total number, they can only know the proportions. It would be great if, given a choice between their answer and one involving twice as many marbles, they could explain that either answer was equally likely.
It would not be good if they guessed the same number as the numbers in the results. But if you keep drawing marbles, eventually they will see the folly of that.
Of course, the other part of the assignment is guessing the proportion. The best answer (probably?! -- approximately??) is to choose a proportion equal to the one that was observed. They might depart a little just for the sake of interest or an inability to accurately express the proportion. Note that if the observed proportion of blacks was 2/7, then 2/7 is probably a better estimate than 1/3. However, if the observed proportion of blacks was 69/100, 1/3 is probably a better guess than 69/100.
There is one more game to play with this assignment. Suppose you pulled out a black marble 20 times and a white marble 10 times, and they have made their guesses. You can then ask how many green marbles there are in the bag. Actually, at this point, 1 is probably a reasonable guess, given that you have just asked about green. But if you keep asking about other colors, eventually they will probably change their estimate to zero, which again is probably the reasonable thing to do (because now the colors aren't special).
Of course, you can play this game several times if you brought in several bags.