The basic problem is this. There is a line down the middle of the paper. The student has to say how the things on the left side are all the same, and how they differ from the things on the right side. To give a trivial example, the left side might contain rectangles, and the right side might contain quadilaterals that are not rectangles. You as teacher might be content with the answer "rectangle", or you might want the student to describe how the rectangles differ from the quadrilaterals.
Uses for these Problems
There are three uses for these problems. The first is just to give a problem in pattern recognition. The second is diagnostic. For example, in one of the problems below, the thing that is the same is the proportion of circles in a clump that are colored in black. Presumably this tests if the student has the concept of proportion.
Third, and perhaps most importantly, these problems can be used to challenge students to express the properties of a known category. This was alluded to above -- the problems can be used as a base for asking the student to define the category of rectangle.
As such, this third function is developing communication skills. It is also functioning like a mathematician -- everyone knows what a rectangle is, only mathematicians care about how it is defined. And finally, it deepens concepts -- you do not know what a circle is until you have struggled with defining it.
So, as always, the Problems First method turns things around. Instead of you the teacher defining rectangle or circle, your student does. But that helps your student learn.
Warm-Ups
The starting problems should give the student familiarity with the format. Ideally, they would be mildly challenging but not so difficult that the student couldn't solve the problem. I overestimated the difficulty of the first two -- most of my third-graders couldn't get them. I don't know why.
| Ashley | William |
| Brianna | Michael |
| Mary | Thomas |
| Jane | George |
| Chicago | London |
| Los Angeles | Paris |
| Philadelphia | Moscow |
| Boston | Toronto |
The above problems used words. The remaining problems were nonverbal. Click on the picture to see a bigger version.
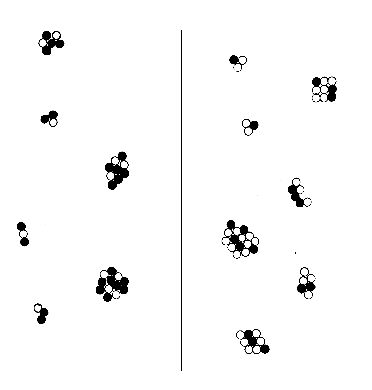
This problem was my first foray into proportions. On the left side, 2/3 of the circles in each clump are colored in; on the right side, 1/3 of the circles in each clump were colored in. Kiana got it. One of my third-graders got it; two did not. This teaches nothing, unless they get it, but it is diagnostic. It warned me that I need to work on the concept of proportion.
This page is currently under construction.
Definitions
Just asking for definitions approaches this problem in the same way. Definitions are not as rigorous at setting up the problem, but they will sometimes work. For an example outside mathematics, students could be asked to define "bottle". This is a very difficult problem, even for an adult. (People have the information, but it is buried in their unconscious in a form that is difficult to retrieve.)
Students will undoubtably be satisfied with their definitions, so it is up to the teacher to point out the problems. Do this by showing that something that is not a bottle (such as a vase or bowl) fills the definition, or something that is a bottle (a plastic bottle) doesn't fit the definition. I guess that gives the teacher the task of explaining the value of the assignment to children who tend to focus on just getting the right answer and will not naturally appreciate the teacher's efforts. "If you actually wanted to be right, it would be useful to make sure that objects that are not bottles don't fit your definition and objects that are bottles do fit the definition."
If you do this enough, you also need to be sensitive to what I call the three-legged dog problem. It is natural to define a dog as a collection of properties -- having a tail, barking, having four legs, etc. Something with most of the properties would still be called a dog, but it will be a dog that doesn't bark, a tailless dog, or a three-legged dog.